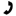If the area and perimeter of a right triangle are 30 cm2 and 30 cm, respectively, what is the length of the hypotenuse (the side opposite the right angle)?

Loading ...
Correct Answer: D. 13
If we denote the hypotenuse of the triangle as c and the two other sides as a and b, then the values of the area and perimeter can be written as (1/2)ab = 30 and a + b + c = 30. We have three unknowns but two equations so far. However, a right triangle also follows the relation a2 + b2 = c2. We can now solve these three equations and obtain c = 13.
However, the “spirit” of the problem is not checking whether you can solve this system of 3 equations (which is pretty complicated!) – instead, this problem is trying to see if you can come up with a “shortcut” to get to the right answer. Since both the perimeter and area are both nice, whole numbers, we can conclude that the lengths of the sides are also nice, whole numbers, most likely from one of the “special” triangles that we know about (eg. 3-4-5, 5-12-13) or a similar triangle. We can immediately see that the sides of the 5-12-13 triangle add up to 30. The area of the 5-12-13 triangle is 30 as well, and we can thus get to the correct answer without doing any solving at all. The work is shown below for your convenience, however.

Subscribe below to get the DAT Question of the Day delivered straight to your inbox every morning.